How to Conquer the Times Table, Part 1

Photo of Evil Erin times 5 (and dog times 2), via flickr.

Perhaps the biggest challenge for any middle-elementary math student is to master the multiplication facts. It can seem like an unending task to memorize so many facts and be able to pull them out of mental storage in any order on demand. Too often, the rote aspect of such memory work overwhelms students, eclipsing their view of the principles behind the math. Yet rote memory is not enough: A student may be able to recite the times tables perfectly and still be reduced to counting on fingers in the middle of a long division problem.

We will use the world’s oldest interactive game — conversation — to learn the multiplication facts one bite at a time. But first, let’s take some time to think about what multiplication really means.

What Is Multiplication?

Before practicing the times table facts, make sure your student understands what multiplication does. Multiplication is like a mad scientist’s ray gun that can enlarge or shrink things according to which scale factor the scientist sets:

[number] × [number] = [scale factor] “times the size of” [original amount]

That is where we get the word times for the multiplication symbol, though I also teach my students to use the word of, especially when multiplying with fractions or percents. If the scientist sets the scale factor at 1.0, that will leave the item exactly the same size. Any number greater than one will make it grow, while a number less than one will shrink it.

5.6 × 3 = 5.6 times the size of 3
4 × 7 = 4 times the size of 7
¼ × 8 = ¼ of 8
30% × 600 = 30% of 600

Ray Gun Control Settings

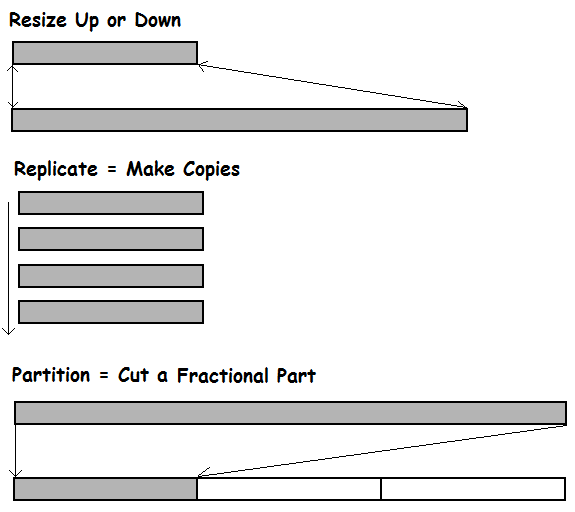
The ray gun has another setting in addition to the scale factor. This setting controls the type of growth or shrinkage. The scientist can make something grow by resizing (changing the size) or by replication (copying). With his Resize setting, he can turn a cockroach into a monster 351⅞ times its original size. Then he can switch to the Replicate setting, which creates multiple copies of the monster, until he has a whole army of giant cockroaches ready to attack. Similarly, the mad scientist can shrink things by resizing or by partitioning (cutting it down to a fractional part).

The Resize setting may be used with any scale factor, but the Replicate setting needs a whole number scale factor (how many copies), and the Partition setting needs a simple fraction or percent scale factor.

Replication is Repeated Addition

The mad scientist’s Replicate setting is like the common textbook description of multiplication as repeatedly adding the same amount. If he fixes six bowls of stew and lines them up in a row, he can use the Replicate setting to create row upon row of additional bowls, until he has more than enough stew to feed all the minions. Our students can use replication to model multiplication with rows of blocks, making an original row and then copying it again and again, until they have as many rows as the scale factor says.

Many Models of Multiplication

To see what multiplication looks like in real life, explore the multitude of Multiplication Models collected at the Natural Math website. Also available as a poster for easy reference.

This is the second post in my Times Table Series. To be continued… [Go to part 2.]